All things being relative, the past few days have been rather uneventful. The winds have been steady and we’ve made good distance, but by a few hours after sunrise this morning, the winds had quieted down and we were just barely moving along.  As you might expect, this stirs up some feelings of frustration, especially when you’ve been out at sea for 7 weeks and there’s still 2500 miles to go!

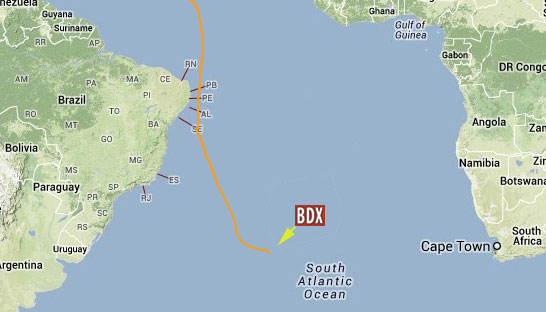29.67041W, 26.92078S – Trust me, Cape Town is farther away than it looks

I don’t know what other distance sailors do, but on days like today, my mind starts to grind out math problems. I’ll spend time catching up on the navigation issues and plotting courses on the charts, but my mind can’t help but work on the overriding question of when I will get to Cape Town.

So, what I thought I’d do with today’s update is something a little different, but still very much in keeping with our overall “learning and discovery” agenda – and that is to plunge into some of these relatively simple time and distance problems that are at the heart of ocean navigation. (Also, look for these kinds of problems too, in our new BDX Explorer Guides, being worked on right now!)

Now I’m no math wizard, mind you, and I don’t have a college degree and like most kids, I often found myself asking my teachers when will I ever use this math stuff? Well, as it turns out, once I took up building homes and sailing, math quickly became THE most useful stuff to know!

When building or renovating homes, you have to constantly calculate costs and quantities. You need to use geometry to figure out how to build square buildings, and how much plywood is needed on a slanted roof.

With sailing, you need to know how far away something is, what angle you need to steer and if you can’t make it on that angle, how much of a “tack” you’re going to have to take to get to where you want to go. (A tack is a change of direction to sail “against” the wind in the other direction … like zig-zagging into the wind.)

So, this morning, with about 300 miles to go to the waypoint and another 2150 miles after that to Cape Town, and the weather forecast calling for a front passing through, just as I get to the waypoint, I sat back and let my internal calculator take over.

Would you like to try some of the problems I worked on? Ok, here goes. Let’s try one with the solution first.

• It’s Monday, at 06:00 hours and I’m 300 miles from the waypoint. The weather front is predicted to arrive on Tuesday evening at about 18:00 hours and if I can arrive about that time, I can take advantage of the good winds to make additional distance toward Cape Town. I’ve been sailing at about 8.5 knots all night long, but once the sun rose, the winds died down and my speed dropped to 5.75 knots. Question then … how many hours will it take me to get to the waypoint? And can I make it before the weather front?
• So, in this first one, the waypoint is 300 miles away – so just take the distance (300) and divide it by the speed (5.75) and that equals a bit more than 52 hours. Then time-wise, from Monday at 6:00 am until Tuesday at 18:00 (6:00 pm)  that’s 36  hours. so, 36 hours multiplied by 5.75 knots equals 207 miles, so you can see I won’t make it.

Let’s try some more!

1. Now, if I use my engine some until the winds come back (usually just after high sun or noon) and can nudge the speed up to 6.5 knots until 12:00 hours and am then able to sail at 6.5 knots the rest of the way, how long will it take me … and can I make it to the waypoint before the front?
2. What speed would I have to average to make it to the waypoint on time?
3. It’s now later in the day, I just passed the 200 mark at 19:45 hours and I have 200 miles to go to the waypoint. The winds have increased nicely and with my biggest sail up, I am sailing along again at 8.5 knots. How close will I be to making the waypoint?
4. If I can make the waypoint by 18:00 hours on Tuesday and there are still 2150 miles from there to Cape Town, and if I average 150 miles a day, what day would I get there? And how many knots per hour do I have to go, to make 150 miles a day?
5. Usually, after a front passes, the winds are pretty fresh and I can sail at pretty good speeds. So, here’s a tougher problem for you. If I reach the waypoint and am able to sail for 60 hours at 8.5 knots before the winds ease back, and if I then sail for 5 days averaging 7 knots an hour, how many miles will be left to Cape Town? If I were able to sail them at 8 miles an hour, how would that change my arrival date in Cape Town?

So, all of the above are time and distance problems, that apply to long distance sailing. Racing and particularly inshore racing around buoys and markers raise other types of math problems. In this case, you need to apply math skills to plan your strategic maneuvers. Let’s try one of those.

• If I know I’m going to need to change sails at a particular mark rounding and I know it will take me 4 minutes to set up the boat to make the sail change, I need to know at what distance we will be at with 4 minutes left to reach the mark. Let’s say we are sailing at 6 knots. We know then that a mile takes 10 minutes to sail. What would my GPS tell me the distance is to the mark at four minutes before we reach it?

Interesting stuff, huh?  I think so, but more than that, it’s an essential part of sailing that people who don’t sail, may not fully appreciate.

Well, there you have it from the ocean … straight from the skipper’s notepad. For so many things, where accuracy is important, math is a necessity to figure out how to do the things you need to do. Once I get to Cape Town, I’ll have to start figuring out all sorts of things for my next leg: the amounts of food to take, fuel, etc. And I aim to do better job of calculating out cookies, so I don’t run out so quickly!

So, until later … from the cookie-less South Atlantic …

-Dave, Bodacious Dream and the always studious Franklin
29.67041W, 26.92078S

To get major updates by email … sign up for our Mailing List!